It is absolutely impossible to solve physical problems or examples in mathematics without knowledge about the derivative and methods for calculating it. The derivative is one of the most important concepts of mathematical analysis. We decided to devote today's article to this fundamental topic. What is a derivative, what is its physical and geometric meaning, how to calculate the derivative of a function? All these questions can be combined into one: how to understand the derivative?

Geometric and physical meaning of the derivative

Let there be a function f(x) , given in some interval (a,b) . The points x and x0 belong to this interval. When x changes, the function itself changes. Argument change - difference of its values x-x0 . This difference is written as delta x and is called argument increment. The change or increment of a function is the difference between the values ​​of the function at two points. Derivative definition:

The derivative of a function at a point is the limit of the ratio of the increment of the function at a given point to the increment of the argument when the latter tends to zero.

Otherwise it can be written like this:

What is the point in finding such a limit? But which one:

the derivative of a function at a point is equal to the tangent of the angle between the OX axis and the tangent to the graph of the function at a given point.

The physical meaning of the derivative: the time derivative of the path is equal to the speed of the rectilinear motion.

Indeed, since school days, everyone knows that speed is a private path. x=f(t) and time t . Average speed over a certain period of time:

To find out the speed of movement at a time t0 you need to calculate the limit:

Rule one: take out the constant

The constant can be taken out of the sign of the derivative. Moreover, it must be done. When solving examples in mathematics, take as a rule - if you can simplify the expression, be sure to simplify .

Example. Let's calculate the derivative:

Rule two: derivative of the sum of functions

The derivative of the sum of two functions is equal to the sum of the derivatives of these functions. The same is true for the derivative of the difference of functions.

We will not give a proof of this theorem, but rather consider a practical example.

Find the derivative of a function:

Rule three: the derivative of the product of functions

The derivative of the product of two differentiable functions is calculated by the formula:

Example: find the derivative of a function:

Solution:

Here it is important to say about the calculation of derivatives of complex functions. The derivative of a complex function is equal to the product of the derivative of this function with respect to the intermediate argument by the derivative of the intermediate argument with respect to the independent variable.

In the above example, we encounter the expression:

In this case, the intermediate argument is 8x to the fifth power. In order to calculate the derivative of such an expression, we first consider the derivative of the external function with respect to the intermediate argument, and then multiply by the derivative of the intermediate argument itself with respect to the independent variable.

Rule Four: The derivative of the quotient of two functions

Formula for determining the derivative of a quotient of two functions:

We tried to talk about derivatives for dummies from scratch. This topic is not as simple as it seems, so be warned: there are often pitfalls in the examples, so be careful when calculating derivatives.

With any question on this and other topics, you can contact the student service. In a short time, we will help you solve the most difficult control and deal with tasks, even if you have never dealt with the calculation of derivatives before.

Function research. In this article, we will talk about tasks in which functions are considered and in the condition there are questions related to their study. Consider the main theoretical points that you need to know and understand to solve them.

This is a whole group of tasks included in the exam in mathematics. The question is usually raised about finding the points of maximum (minimum) or determining the largest (smallest) value of a function on a given interval.Considered:

— Power and irrational functions.

— Rational functions.

— Study of works and private.

— Logarithmic functions.

— Trigonometric functions.

If you understand the theory of limits, the concept of a derivative, the properties of a derivative for studying graphs of functions and its , then such problems will not cause you any difficulty and you will solve them with ease.

The information below is theoretical points, the understanding of which will make it possible to realize how to solve such problems. I will try to state them in such a way that even those who missed this topic or studied it poorly could solve such problems without much difficulty.

In the problems of this group, as already mentioned, it is required to find either the minimum (maximum) point of the function, or the largest (smallest) value of the function on the interval.

Minimum and maximum points.Derivative properties.

Consider the graph of the function:

Point A is the maximum point, on the interval from O to A the function increases, on the interval from A to B it decreases.

Point B is a minimum point, on the interval from A to B the function decreases, on the interval from B to C it increases.

At these points (A and B), the derivative vanishes (equals to zero).

The tangents at these points are parallel to the axis ox.

I will add that the points at which the function changes its behavior from increasing to decreasing (and vice versa, from decreasing to increasing) are called extrema.

Important point:

1. The derivative on increasing intervals has a positive sign (nWhen substituting a value from the interval into the derivative, a positive number is obtained).

So, if the derivative at a certain point from a certain interval has a positive value, then the graph of the function on this interval increases.

2. On the intervals of decreasing, the derivative has a negative sign (when substituting the value from the interval into the derivative expression, a negative number is obtained).

So, if the derivative at a certain point from a certain interval has a negative value, then the graph of the function on this interval decreases.

This needs to be made clear!

Thus, by calculating the derivative and equating it to zero, you can find points that divide the real axis into intervals.On each of these intervals, you can determine the sign of the derivative and then draw a conclusion about its increase or decrease.

* Separately, it should be said about the points at which the derivative does not exist. For example, we can get a derivative whose denominator vanishes at a certain x. It is clear that for such x the derivative does not exist. So, this point must also be taken into account when determining the intervals of increase (decrease).

The function at points where the derivative is equal to zero does not always change its sign. This will be a separate article. There will be no such tasks at the USE itself.

The above properties are necessary to study the behavior of a function in increasing and decreasing.

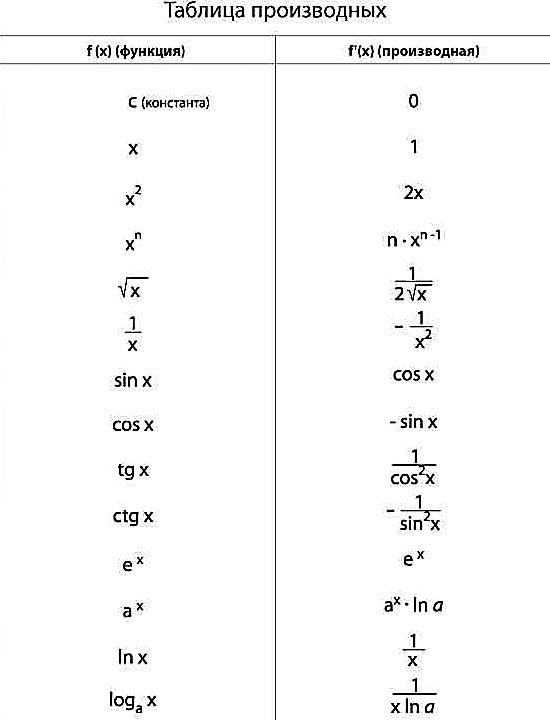
What else you need to know to solve the specified problems: the table of derivatives and the rules of differentiation. Nothing without this. This is basic knowledge in the topic of derivative. You should know the derivatives of elementary functions very well.

Calculating the derivative of a complex functionf(g(x)), imagine the functiong(x) is a variable and then calculate the derivativef’(g(x)) by tabular formulas as an ordinary derivative of a variable. Then multiply the result by the derivative of the functiong(x) .

Watch a video tutorial by Maxim Semenikhin about a complex function:

Problems for finding maximum and minimum points

The algorithm for finding the maximum (minimum) points of the function:

1. Find the derivative of the function f’(x).

2. Find the zeros of the derivative (by equating the derivative to zero f’(x)=0 and solve the resulting equation). We also find points where the derivative does not exist(in particular, this concerns fractional-rational functions).

3. We mark the obtained values ​​on the number line and determine the signs of the derivative on these intervals by substituting the values ​​from the intervals into the derivative expression.

The output will be one of two:

1. The maximum point is the pointin which the derivative changes from positive to negative.

2. The minimum point is the pointin which the derivative changes from negative to positive.

Problems for finding the largest or smallest value

functions on the interval.

In another type of problem, it is required to find the largest or smallest value of a function on a given interval.

The algorithm for finding the largest (smallest) function value:

1. Determine if there are maximum (minimum) points. To do this, we find the derivative f’(x) , then solve f’(x)=0 (points 1 and 2 from the previous algorithm).

2. We determine whether the obtained points belong to a given interval and write down those lying within it.

3. We substitute into the original function (not into the derivative, but into the given one in the condition) the boundaries of the given interval and the points (maximum-minimum) lying within the interval (item 2).

4. We calculate the values ​​of the function.

5. We select the largest (smallest) value from the obtained ones, depending on what question was posed in the task, and then write down the answer.

Question: why in the tasks of finding the largest (smallest) value of a function, it is necessary to look for maximum (minimum) points?

The answer is best illustrated, see a schematic representation of the graphs given by the functions:

In cases 1 and 2, it is enough to substitute the boundaries of the interval to determine the maximum or minimum value of the function. In cases 3 and 4, it is necessary to find the zeros of the function (maximum-minimum points). If we substitute the boundaries of the interval (without finding the zeros of the function), we will get the wrong answer, this can be seen from the graphs.

And the thing is that we cannot see how the chart looks on the interval (whether it has a maximum or a minimum within the interval) using a given function. Therefore, find the zeros of the function without fail!!!

If the equation f'(x)=0 will not have a solution, this means that there are no maximum-minimum points (Figure 1.2), and in order to find the set task, only the boundaries of the interval are substituted into this function.

Another important point. Remember that the answer must be an integer or a final decimal. When calculating the largest and smallest value of a function, you will receive expressions with the number e and pi, as well as expressions with a root. Remember that you do not need to calculate them to the end, and it is clear that the result of such expressions will not be the answer. If there is a desire to calculate such a value, then do it (numbers: e ≈ 2.71 Pi ≈ 3.14).

I wrote a lot, probably confused? By specific examples, you will see that everything is simple.

Next, I want to tell you a little secret. The fact is that many tasks can be solved without knowing the properties of the derivative and even without the rules of differentiation. I will definitely tell you about these nuances and show you how it is done? do not miss!

But then why did I state the theory at all and also said that it must be known without fail. That's right - you need to know. If you understand it, then no task in this topic will confuse you.

Those “tricks” that you will learn about will help you in solving specific (some) prototype problems. ToAs an additional tool, these techniques are, of course, convenient to use. The problem can be solved 2-3 times faster and save time for solving part C.

All the best!

Sincerely, Alexander Krutitskikh.

P.S: I would be grateful if you tell me about the site on social networks.

Derivative of a function of one variable.

Introduction.

These methodological developments are intended for students of the Faculty of Industrial and Civil Engineering. They are compiled in relation to the program of the course of mathematics in the section "Differential calculus of functions of one variable."

The developments represent a single methodological guide, which includes: brief theoretical information; "typical" tasks and exercises with detailed solutions and explanations for these solutions; control options.

Additional exercises at the end of each paragraph. Such a structure of developments makes them suitable for independent mastering of the section with the most minimal assistance from the teacher.

§one. Definition of a derivative.

Mechanical and geometric meaning

derivative.

The concept of a derivative is one of the most important concepts in mathematical analysis. It arose as early as the 17th century. The formation of the concept of a derivative is historically associated with two problems: the problem of the speed of variable motion and the problem of a tangent to a curve.

These tasks, despite their different content, lead to the same mathematical operation that must be performed on a function. This operation has received a special name in mathematics. It is called the operation of differentiating a function. The result of a differentiation operation is called a derivative.

So, the derivative of the function y=f(x) at the point x0 is the limit (if it exists) of the ratio of the increment of the function to the increment of the argument
at
.

The derivative is usually denoted as follows:
.

So by definition

The symbols are also used to denote the derivative
.

The mechanical meaning of the derivative.

If s=s(t) is the law of rectilinear motion of a material point, then
is the speed of this point at time t.

The geometric meaning of the derivative.

If the function y=f(x) has a derivative at a point , then the slope of the tangent to the graph of the function at the point
equals
.

Example.

Find the derivative of a function
at the point =2:

1) Let's give a point =2 increment
. Notice, that.

2) Find the increment of the function at the point =2:

3) Compose the ratio of the increment of the function to the increment of the argument:

Let us find the limit of the relation at
:

.

In this way,
.

§ 2. Derivatives of some

the simplest functions.

The student needs to learn how to calculate the derivatives of specific functions: y=x,y= and in general y= .

Find the derivative of the function y=x.

those. (x)′=1.

Let's find the derivative of the function

Derivative

Let
then

It is easy to notice a pattern in the expressions for derivatives of a power function
at n=1,2,3.

Consequently,

. (1)

This formula is valid for any real n.

In particular, using formula (1), we have:

;

.

Example.

Find the derivative of a function

.

.

This function is a special case of a function of the form

at
.

Using formula (1), we have

.

Derivatives of functions y=sin x and y=cos x.

Let y=sinx.

Divide by ∆x, we get

Passing to the limit as ∆x→0, we have

Let y=cosx .

Passing to the limit as ∆x→0, we obtain

;
. (2)

§3. Basic rules of differentiation.

Consider the rules of differentiation.

Theorem1 . If the functions u=u(x) and v=v(x) are differentiable at a given point x, then their sum is also differentiable at this point, and the derivative of the sum is equal to the sum of the derivative terms: (u+v)"=u"+v".(3 )

Proof: consider the function y=f(x)=u(x)+v(x).

The increment ∆x of the argument x corresponds to the increments ∆u=u(x+∆x)-u(x), ∆v=v(x+∆x)-v(x) of the functions u and v. Then the function y will be incremented

∆y=f(x+∆x)-f(x)=

=--=∆u+∆v.

Consequently,

So, (u+v)"=u"+v".

Theorem2. If the functions u=u(x) and v=v(x) are differentiable at a given point x, then their product is also differentiable at the same point. In this case, the derivative of the product is found by the following formula: (uv) "=u" v + uv ". ( four)

Proof: Let y=uv, where u and v are some differentiable functions of x. Let x be incremented by ∆x; then u will be incremented by ∆u, v will be incremented by ∆v, and y will be incremented by ∆y.

We have y+∆y=(u+∆u)(v+∆v), or

y+∆y=uv+u∆v+v∆u+∆u∆v.

Therefore, ∆y=u∆v+v∆u+∆u∆v.

From here

Passing to the limit as ∆x→0 and taking into account that u and v do not depend on ∆x, we have

Theorem 3. The derivative of a quotient of two functions is equal to a fraction, the denominator of which is equal to the square of the divisor, and the numerator is the difference between the product of the derivative of the dividend by the divisor and the product of the dividend by the derivative of the divisor, i.e.

If a
then
(5)

Theorem 4. The derivative of the constant is zero, i.e. if y=C, where С=const, then y"=0.

Theorem 5. The constant factor can be taken out of the sign of the derivative, i.e. if y=Cu(x), where С=const, then y"=Cu"(x).

Example 1

Find the derivative of a function

.

This function has the form
, where u=x,v=cosx. Applying the differentiation rule (4), we find

.

Example 2

Find the derivative of a function

.

We apply formula (5).

Here
;
.

Tasks.

Find derivatives of the following functions:

;

11)

2)
; 12)
;

3)
13)

4)
14)

5)
15)

6)
16)

7 )
17)

8)
18)

9)
19)

10)
20)

Compose the ratio and calculate the limit.

Where did table of derivatives and differentiation rules? Thanks to a single limit. It seems like magic, but in reality - sleight of hand and no fraud. On the lesson What is a derivative? I began to consider specific examples, where, using the definition, I found the derivatives of a linear and quadratic function. For the purpose of cognitive warm-up, we will continue to disturb derivative table, honing the algorithm and technical solutions:

Example 1

In fact, it is required to prove a special case of the derivative of a power function, which usually appears in the table: .

Solution technically formalized in two ways. Let's start with the first, already familiar approach: the ladder starts with a plank, and the derivative function starts with a derivative at a point.

Consider some(specific) point belonging to domains a function that has a derivative. Set the increment at this point (of course, not beyondo/o -I) and compose the corresponding increment of the function:

Let's calculate the limit:

Uncertainty 0:0 is eliminated by a standard technique considered as far back as the first century BC. Multiply the numerator and denominator by the adjoint expression :

The technique for solving such a limit is discussed in detail in the introductory lesson. about the limits of functions.

Since ANY point of the interval can be chosen as, then, by replacing , we get:

Answer

Once again, let's rejoice at the logarithms:

Example 2

Find the derivative of the function using the definition of the derivative

Solution: let's consider a different approach to the promotion of the same task. It is exactly the same, but more rational in terms of design. The idea is to get rid of the subscript at the beginning of the solution and use the letter instead of the letter .

Consider arbitrary point belonging to domains function (interval ), and set the increment in it. And here, by the way, as in most cases, you can do without any reservations, since the logarithmic function is differentiable at any point in the domain of definition.

Then the corresponding function increment is:

Let's find the derivative:

The ease of design is balanced by the confusion that beginners (and not only) can experience. After all, we are used to the fact that the letter “X” changes in the limit! But here everything is different: - an antique statue, and - a living visitor, cheerfully walking along the corridor of the museum. That is, “x” is “like a constant”.

I will comment on the elimination of uncertainty step by step:

(1) Use the property of the logarithm .

(2) In brackets, we divide the numerator by the denominator term by term.

(3) In the denominator, we artificially multiply and divide by "x" to take advantage of wonderful limit , while as infinitesimal stands out.

Answer: by definition of derivative:

Or in short:

I propose to independently construct two more tabular formulas:

Example 3

In this case, the compiled increment is immediately convenient to reduce to a common denominator. An approximate sample of the assignment at the end of the lesson (the first method).

Example 3:Solution : consider some point , belonging to the scope of the function . Set the increment at this point and compose the corresponding increment of the function:

Let's find the derivative at a point :


Since as you can choose any point function scope , then and
Answer : by definition of the derivative

Example 4

Find derivative by definition

And here everything must be reduced to wonderful limit. The solution is framed in the second way.

Similarly, a number of other tabular derivatives. A complete list can be found in a school textbook, or, for example, the 1st volume of Fichtenholtz. I don’t see much point in rewriting from books and proofs of the rules of differentiation - they are also generated by the formula.

Example 4:Solution , owned , and set an increment in it

Let's find the derivative:

Making use of the wonderful limit

Answer : by definition

Example 5

Find the derivative of a function , using the definition of the derivative

Solution: Use the first visual style. Let's consider some point belonging to , let's set the increment of the argument in it. Then the corresponding function increment is:

Perhaps some readers have not yet fully understood the principle by which an increment should be made. We take a point (number) and find the value of the function in it: , that is, into the function instead of"x" should be substituted. Now we also take a very specific number and also substitute it into the function instead of"x": . We write down the difference , while it is necessary parenthesize completely.

Composed Function Increment it is beneficial to immediately simplify. What for? Facilitate and shorten the solution of the further limit.

We use formulas, open brackets and reduce everything that can be reduced:

The turkey is gutted, no problem with the roast:

Eventually:

Since any real number can be chosen as the quality, we make the substitution and get .

Answer: by definition.

For verification purposes, we find the derivative using differentiation rules and tables:

It is always useful and pleasant to know the correct answer in advance, so it is better to mentally or on a draft differentiate the proposed function in a “quick” way at the very beginning of the solution.

Example 6

Find the derivative of a function by the definition of the derivative

This is a do-it-yourself example. The result lies on the surface:

Example 6:Solution : consider some point , owned , and set the increment of the argument in it . Then the corresponding function increment is:


Let's calculate the derivative:


In this way:
Because as any real number can be chosen and
Answer : by definition.

Let's go back to style #2:

Example 7

Let's find out immediately what should happen. By the rule of differentiation of a complex function:

Solution: consider an arbitrary point belonging to , set the increment of the argument in it and compose the increment of the function:

Let's find the derivative:


(1) Use trigonometric formula .

(2) Under the sine we open the brackets, under the cosine we present similar terms.

(3) Under the sine we reduce the terms, under the cosine we divide the numerator by the denominator term by term.

(4) Due to the oddness of the sine, we take out the “minus”. Under the cosine, we indicate that the term .

(5) We artificially multiply the denominator to use first wonderful limit. Thus, the uncertainty is eliminated, we comb the result.

Answer: by definition

As you can see, the main difficulty of the problem under consideration rests on the complexity of the limit itself + a slight originality of packing. In practice, both methods of design are encountered, so I describe both approaches in as much detail as possible. They are equivalent, but still, in my subjective impression, it is more expedient for dummies to stick to the 1st option with “X zero”.

Example 8

Using the definition, find the derivative of the function

Example 8:Solution : consider an arbitrary point , owned , let's set an increment in it and make an increment of the function:

Let's find the derivative:

We use the trigonometric formula and the first remarkable limit:

Answer : by definition

Let's analyze a rarer version of the problem:

Example 9

Find the derivative of a function at a point using the definition of a derivative.

First, what should be the bottom line? Number

Let's calculate the answer in the standard way:

Solution: from the point of view of clarity, this task is much simpler, since the formula considers a specific value instead.

We set an increment at the point and compose the corresponding increment of the function:

Calculate the derivative at a point:

We use a very rare formula for the difference of tangents and once again reduce the solution to first wonderful limit:

Answer: by definition of the derivative at a point.

The task is not so difficult to solve and “in general terms” - it is enough to replace with or simply, depending on the design method. In this case, of course, you get not a number, but a derivative function.

Example 10

Using the definition, find the derivative of the function at a point (one of which may turn out to be infinite), which I have already talked about in general terms on theoretical lesson about the derivative.

Some piecewise defined functions are also differentiable at the “junction” points of the graph, for example, catdog has a common derivative and a common tangent (abscissa) at the point . Curve, yes differentiable by ! Those who wish can verify this for themselves on the model of the just solved example.

©2015-2019 site
All rights belong to their authors. This site does not claim authorship, but provides free use.
Page creation date: 2017-06-11

Job type: 7

Condition

The line y=3x+2 is tangent to the graph of the function y=-12x^2+bx-10. Find b , given that the abscissa of the touch point is less than zero.

Show Solution

Solution

Let x_0 be the abscissa of the point on the graph of the function y=-12x^2+bx-10 through which the tangent to this graph passes.

The value of the derivative at the point x_0 is equal to the slope of the tangent, i.e. y"(x_0)=-24x_0+b=3. On the other hand, the tangent point belongs to both the graph of the function and the tangent, i.e. -12x_0^2+bx_0-10= 3x_0 + 2. We get a system of equations \begin(cases) -24x_0+b=3,\\-12x_0^2+bx_0-10=3x_0+2. \end(cases)

Solving this system, we get x_0^2=1, which means either x_0=-1 or x_0=1. According to the condition of the abscissa, the touch points are less than zero, therefore x_0=-1, then b=3+24x_0=-21.

Answer

Job type: 7
Topic: The geometric meaning of the derivative. Tangent to function graph

Condition

The line y=-3x+4 is parallel to the tangent to the graph of the function y=-x^2+5x-7. Find the abscissa of the point of contact.

Show Solution

Solution

The slope of the line to the graph of the function y=-x^2+5x-7 at an arbitrary point x_0 is y"(x_0). But y"=-2x+5, so y"(x_0)=-2x_0+5. Angular the coefficient of the line y=-3x+4 specified in the condition is -3.Parallel lines have the same slopes.Therefore, we find such a value x_0 that =-2x_0 +5=-3.

We get: x_0 = 4.

Answer

Source: "Mathematics. Preparation for the exam-2017. profile level. Ed. F. F. Lysenko, S. Yu. Kulabukhova.

Job type: 7
Topic: The geometric meaning of the derivative. Tangent to function graph

Condition

Show Solution

Solution

From the figure, we determine that the tangent passes through the points A(-6; 2) and B(-1; 1). Denote by C(-6; 1) the point of intersection of the lines x=-6 and y=1, and by \alpha the angle ABC (it can be seen in the figure that it is sharp). Then the line AB forms an obtuse angle \pi -\alpha with the positive direction of the Ox axis.

As you know, tg(\pi -\alpha) will be the value of the derivative of the function f(x) at the point x_0. notice, that tg \alpha =\frac(AC)(CB)=\frac(2-1)(-1-(-6))=\frac15. From here, by the reduction formulas, we obtain: tg(\pi -\alpha) =-tg \alpha =-\frac15=-0.2.

Answer

Source: "Mathematics. Preparation for the exam-2017. profile level. Ed. F. F. Lysenko, S. Yu. Kulabukhova.

Job type: 7
Topic: The geometric meaning of the derivative. Tangent to function graph

Condition

The line y=-2x-4 is tangent to the graph of the function y=16x^2+bx+12. Find b , given that the abscissa of the touch point is greater than zero.

Show Solution

Solution

Let x_0 be the abscissa of the point on the graph of the function y=16x^2+bx+12 through which

is tangent to this graph.

The value of the derivative at the point x_0 is equal to the slope of the tangent, i.e. y "(x_0)=32x_0+b=-2. On the other hand, the tangent point belongs to both the graph of the function and the tangent, i.e. 16x_0^2+bx_0+12=- 2x_0-4 We get a system of equations \begin(cases) 32x_0+b=-2,\\16x_0^2+bx_0+12=-2x_0-4. \end(cases)

Solving the system, we get x_0^2=1, which means either x_0=-1 or x_0=1. According to the condition of the abscissa, the touch points are greater than zero, therefore x_0=1, then b=-2-32x_0=-34.

Answer

Source: "Mathematics. Preparation for the exam-2017. profile level. Ed. F. F. Lysenko, S. Yu. Kulabukhova.

Job type: 7
Topic: The geometric meaning of the derivative. Tangent to function graph

Condition

The figure shows a graph of the function y=f(x) defined on the interval (-2; 8). Determine the number of points where the tangent to the graph of the function is parallel to the straight line y=6.

Show Solution

Solution

The line y=6 is parallel to the Ox axis. Therefore, we find such points at which the tangent to the function graph is parallel to the Ox axis. On this chart, such points are extremum points (maximum or minimum points). As you can see, there are 4 extremum points.

Answer

Source: "Mathematics. Preparation for the exam-2017. profile level. Ed. F. F. Lysenko, S. Yu. Kulabukhova.

Job type: 7
Topic: The geometric meaning of the derivative. Tangent to function graph

Condition

The line y=4x-6 is parallel to the tangent to the graph of the function y=x^2-4x+9. Find the abscissa of the point of contact.

Show Solution

Solution

The slope of the tangent to the graph of the function y \u003d x ^ 2-4x + 9 at an arbitrary point x_0 is y "(x_0). But y" \u003d 2x-4, which means y "(x_0) \u003d 2x_0-4. The slope of the tangent y \u003d 4x-7 specified in the condition is equal to 4. Parallel lines have the same slopes. Therefore, we find such a value x_0 that 2x_0-4 \u003d 4. We get: x_0 \u003d 4.

Answer

Source: "Mathematics. Preparation for the exam-2017. profile level. Ed. F. F. Lysenko, S. Yu. Kulabukhova.

Job type: 7
Topic: The geometric meaning of the derivative. Tangent to function graph

Condition

The figure shows the graph of the function y=f(x) and the tangent to it at the point with the abscissa x_0. Find the value of the derivative of the function f(x) at the point x_0.

Show Solution

Solution

From the figure, we determine that the tangent passes through the points A(1; 1) and B(5; 4). Denote by C(5; 1) the point of intersection of the lines x=5 and y=1, and by \alpha the angle BAC (it can be seen in the figure that it is acute). Then the line AB forms an angle \alpha with the positive direction of the Ox axis.