6th grade
SUBJECT: "Division of ordinary fractions", grade 6.
THE PURPOSE OF THE LESSON: Summarize and systematize theoretical and practical
knowledge, skills and abilities of students. Organize work for
filling gaps in students' knowledge. improve, expand
and deepen students' knowledge of the topic.
LESSON TYPE: Lesson of generalization and systematization of knowledge, skills and abilities.
Equipment: On the board is the topic, goal, lesson plan.
DURING THE CLASSES.
Each student has a checklist on their desk.
1. homework -
2. revision questions -
3. verbal account -
4. class work -
5. independent work -
1. Checking homework:
a) work in pairs on the following questions:
1) Addition, subtraction of ordinary fractions;
2) How to multiply a fraction by a fraction;
3) Multiplication of two fractions;
4) Multiplication of mixed fractions;
5) The rule for dividing fractions;
6) Division of mixed fractions;
7) What is called. reduction of fractions.
b) checking homework according to the finished solution on the board:
No. 620 (a), 624, 619 (d).
Purpose: to determine the degree of assimilation of homework. Identify common weaknesses.
Put the grades on the control sheet
Announce the purpose of the lesson: To generalize and systematize knowledge, skills and abilities in
topic: "Division of ordinary fractions."
The theory was repeated, we will check the knowledge in practice.
2. Verbal counting.
a) On cards: 1) Reduce the fraction:; ; ; …
2) Convert to an improper fraction: ; ; …
3) Select the integer part: ; ; …
b) Numerical ladder. Whoever gets to the 6th floor faster will know:
construction of geometry (Euclid)
Option 2 - a person who wanted to be a lawyer, an officer, and a philosopher, but
became a mathematician (Descartes)
l 0.1: ½ 0.4: 0.1 a
i d e l k c a v r e t
Grades in the control sheet, for: 2 "-"5", 3" - "4", 4" - "3".
Whoever completed the “ladder” does No. 606 in notebooks. The first of the students on the wing of the board does No. 606. Then he checks the class.
3.
a) No. 581 (b, d), 587 (with commentary), 591 (l, m, j), 600, 602, 593 (d, c, e, i)
The assignment is done in notebooks and on the board.
b) solve the problem: A thousand rubles were paid for a kg of sweets. How much are
Kg of such sweets?
4.
№ 1 . Run actions:
: answers: 1) 2) 3) 4) .
№ 2 . Represent a fraction as an ordinary fraction and do the following:
0.375: answers: 1) 2) 3) 4)
№ 3 . Solve the equation: answers: 1) 2) 3) 4) 2
№ 4 . On the first day, the tourist walked the whole way, and on the second day, the rest. In
how many times more is the part of the road covered by the tourist on the first day than on the
second? Answers: 1) 2) 5 3) 4)
№ 5. Present as a fraction:
: answer: 1) 2) 3) 4)
Check the solution according to the template: No. 1 -4; No. 2 - 1; No. 3 - 4; No. 4 - 4; No. 5 - 3.
Put the grades on the control sheet.
Collect checklists. To sum up. Announce grades for the lesson.
5. Lesson summary:
What ground rules did we repeat today?
6. Homework:
No. 619 (c), 620 (b), 627, individual task No. 617 (a, e, g).
Download:
Preview:
MOU "Gymnasium No. 7"
Torzhok, Tver region
OPEN LESSON ON THE TOPIC:
"DIVISION OF ORDINARY FRACTIONS"
6th grade
Open lesson at the city municipality of Torzhok
(attestation, 2001)
Mathematics teacher: Ufimtseva N.A.
2001
SUBJECT : " Division of ordinary fractions, 6th grade.
THE PURPOSE OF THE LESSON : Summarize and systematize theoretical and practical
Knowledge, skills and abilities of students. Organize work for
Filling gaps in students' knowledge. improve, expand
And to deepen students' knowledge on the topic.
LESSON TYPE : Lesson of generalization and systematization of knowledge, skills and abilities.
Equipment : On the board is the topic, goal, lesson plan.
DURING THE CLASSES.
Each student has a checklist on their desk.
- homework -
- repetition questions -
- verbal counting -
- class work -
- independent work -
- Checking homework:
A) work in pairs on the following questions:
1) Addition, subtraction of ordinary fractions;
2) How to multiply a fraction by a fraction;
3) Multiplication of two fractions;
4) Multiplication of mixed fractions;
5) The rule for dividing fractions;
6) Division of mixed fractions;
7) What is called. reduction of fractions.
B) checking homework according to the finished solution on the board:
No. 620 (a), 624, 619 (d).
Target : to determine the degree of assimilation of homework. Identify common weaknesses.
Put the grades on the control sheet
Announce the purpose of the lesson: To generalize and systematize knowledge, skills and abilities in
Topic: "Division of ordinary fractions."
The theory was repeated, we will check the knowledge in practice.
- Verbal counting.
A) On cards: 1) Reduce the fraction:; ; ; …
2) Convert to an improper fraction: ; ; …
3) Select the integer part: ; ; …
B) Numerical ladder. Whoever gets to the 6th floor faster will know:
Constructions of geometry (Euclid)
Option 2 - a person who wanted to be a lawyer, an officer, and a philosopher, but
Became a mathematician (Descartes)
D t
I p
L 0.1: ½ 0.4: 0.1 a
K to
In e
E d
3 2 4 5
I d d e l k c a v r e t
Grades in the control sheet, for: 2 "-"5", 3" - "4", 4" - "3".
Whoever completed the “ladder” does No. 606 in notebooks. The first of the students on the wing of the board does No. 606. Then he checks the class.
- Repetition and systematization of the main theoretical provisions:
a) No. 581 (b, d), 587 (with commentary), 591 (l, m, j), 600, 602, 593 (d, c, e, i)
The assignment is done in notebooks and on the board.
B) solve the problem: A thousand rubles were paid for a kg of sweets. How much are
Kg of such sweets?
- Independent work. Purpose: to check the mastery of this topic.
№ 1 . Run actions:
: answers: 1) 2) 3) 4) .
№ 2 . Represent a fraction as an ordinary fraction and do the following:
0.375: answers: 1) 2) 3) 4)
№ 3 . Solve the equation: answers: 1) 2) 3) 4) 2
№ 4 . On the first day, the tourist walked the whole way, and on the second day, the rest. In
How many times more is the part of the road covered by the tourist on the first day than on the
Second? Answers: 1) 2) 5 3) 4)
№ 5. Present as a fraction:
: answer: 1) 2) 3) 4)
Check the solution according to the template: No. 1 -4; No. 2 - 1; No. 3 - 4; No. 4 - 4; No. 5 - 3.
Put the grades on the control sheet.
Collect checklists. To sum up. Announce grades for the lesson.
- Lesson summary:
What ground rules did we repeat today?
- Homework:
No. 619 (c), 620 (b), 627, individual task No. 617 (a, e, g)
COURSE WORK
ON ALGEBRA AND PRINCIPLES OF ANALYSIS
ON THIS TOPIC
"TRIGONOMETRIC FUNCTIONS"
Creative group of the department of mathematicians
"Gymnasium No. 3", Udomlya.
Lesson #3-4 designed by the math teacher
Ufimtseva N.A.
2000
MOU "Gymnasium No. 7"
Torzhok, Tver region
PUBLIC LESSON
Class: 6
Presentation for the lesson
















 Back forward
Back forward
Attention! The slide preview is for informational purposes only and may not represent the full extent of the presentation. If you are interested in this work, please download the full version.
Lesson Objectives:
Educational aspect:
- repeat and deepen knowledge on the topic “Division of ordinary fractions”
Development aspect:
- develop the skills of analysis, comparison of material;
- develop attention, memory, speech, logical thinking, independence;
- to promote the development of skills to carry out self-assessment of educational activities.
Educational aspect:
- to instill in students the skill of independence in work, to teach diligence, accuracy;
- educate the need to evaluate their own activities and the work of classmates;
- to cultivate a culture of speech, attention to the accuracy of wording.
Forms of organization of educational activities:
- frontal, individual, game
Used technologies:
- cooperation technology;
- information Technology;
- gaming technologies.
Equipment:
- a computer;
- multimedia projector;
- Microsoft Office PowerPoint presentation;
- task cards
During the classes
I. Organizational moment
II. Verbal counting
1. Calculate the values of expressions, collect the puzzle.

Teacher: Guys, do you recognize what is shown in this photo?
Usolye Sibirskoye is one of the oldest cities in the Angara region, it was founded as a settlement in 1669 thanks to the conquerors of the Siberian expanses, the Yenisei Cossacks, the Mikhalev brothers, who discovered a salt spring on the banks of the Angara River and built a salt pan
2. Without performing any action, compare the quotient with the dividend:
III. Repetition of previously studied material
1. Express a decimal as a fraction. In the table, enter the letters corresponding to the answers found (work in pairs).
0.4 - A 1.2 - R 0.006 - P 3.6 - And 0.9 - Z 5.008 - T 0.05 - U 2.16 - O 0.37 - D 4.44 - C 5.08 - K 2.15 - M
The name of the city of Irkutsk comes from the Irkut River, which flows into the Angara. The city starts from the first Irkutsk prison, founded by the Cossacks under the leadership of Yakov Pokhabov on July 6, 1661. By September 1670, a fortress with four towers was built on the site of the prison, called the Kremlin. Irkutsk almost from the very foundation was the most important stronghold for trade with China. All Russian-Chinese trade caravans passed through the city.
2. Write a common fraction as a decimal. Arrange the resulting numbers in ascending order and read the word (on your own, with subsequent verification).
Answers: 0.8; 0.5; 0.25; 0.12; 0.032; 0.07, the word is Baikal (hyperlink to the unified collection of the DER).
IV. Consolidation of the studied material
1. Fill in the blanks:
1) ![]() ;
;
2) ![]() ;
;
3) ![]() ;
;
4) ![]()
2. The game "Lotto" (students need to solve the first example, then go to the example that starts with the number obtained when solving the previous one, make a sentence).
| I option | II option | ||||
at the source |
|||||
lichen |
coated |
||||
Answers: Rock Shamanka - marble covered with red lichen;
Shaman-stone - a rock lying at the source of the Angara.
V. Physical education
Hands at the sides, arms - wider.
One two three four.
Now we decided to jump.
One two three four.
Stretched - higher, higher ...
We squat - lower, lower.
Get up - sit down...
Get up - sit down...
And now they sat down at the desks.
VI. The solution of the problem
Solve a problem: two cars drove simultaneously towards each other from the cities of Usolye-Sibirskoe and Irkutsk, the distance between which is 80 km. The speed of the first car is the speed of the second. Find the speeds of each car if they meet after forty minutes.
Let be x (km/h)- speed of the second car
Then x (km/h)- speed of the first car
x+ x (km/h)- approach speed
Knowing that the cars met through h and drove together 80 km, let's make an equation:
(x+X) * =80
(x+X) =80:
x=120:1
Answer:
- 1 option FRY
- Option 2 OMUL
VIII. Homework
Compose a task
Last time we learned how to add and subtract fractions (see the lesson "Addition and subtraction of fractions"). The most difficult moment in those actions was bringing fractions to a common denominator.
Now it's time to deal with multiplication and division. The good news is that these operations are even easier than addition and subtraction. To begin with, consider the simplest case, when there are two positive fractions without a distinguished integer part.
To multiply two fractions, you need to multiply their numerators and denominators separately. The first number will be the numerator of the new fraction, and the second will be the denominator.
To divide two fractions, you need to multiply the first fraction by the "inverted" second.
Designation:
From the definition it follows that the division of fractions is reduced to multiplication. To flip a fraction, just swap the numerator and denominator. Therefore, the entire lesson we will consider mainly multiplication.
As a result of multiplication, a reduced fraction can arise (and often does arise) - of course, it must be reduced. If, after all the reductions, the fraction turned out to be incorrect, the whole part should be distinguished in it. But what exactly will not happen with multiplication is reduction to a common denominator: no crosswise methods, maximum factors and least common multiples.
By definition we have:

Multiplication of fractions with an integer part and negative fractions
If there is an integer part in the fractions, they must be converted to improper ones - and only then multiplied according to the schemes outlined above.
If there is a minus in the numerator of a fraction, in the denominator or in front of it, it can be taken out of the limits of multiplication or removed altogether according to the following rules:
- Plus times minus gives minus;
- Two negatives make an affirmative.
Until now, these rules have only been encountered when adding and subtracting negative fractions, when it was required to get rid of the whole part. For a product, they can be generalized in order to “burn” several minuses at once:
- We cross out the minuses in pairs until they completely disappear. In an extreme case, one minus can survive - the one that did not find a match;
- If there are no minuses left, the operation is completed - you can start multiplying. If the last minus is not crossed out, since it did not find a pair, we take it out of the limits of multiplication. You get a negative fraction.
Task. Find the value of the expression:
We translate all fractions into improper ones, and then we take out the minuses outside the limits of multiplication. What remains is multiplied according to the usual rules. We get:

Let me remind you once again that the minus that comes before a fraction with a highlighted integer part refers specifically to the entire fraction, and not just to its integer part (this applies to the last two examples).
Also pay attention to negative numbers: when multiplied, they are enclosed in brackets. This is done in order to separate the minuses from the multiplication signs and make the whole notation more accurate.
Reducing fractions on the fly
Multiplication is a very laborious operation. The numbers here are quite large, and to simplify the task, you can try to reduce the fraction even more before multiplication. Indeed, in essence, the numerators and denominators of fractions are ordinary factors, and, therefore, they can be reduced using the basic property of a fraction. Take a look at the examples:
Task. Find the value of the expression:
By definition we have:

In all examples, the numbers that have been reduced and what is left of them are marked in red.
Please note: in the first case, the multipliers were reduced completely. Units remained in their place, which, generally speaking, can be omitted. In the second example, it was not possible to achieve a complete reduction, but the total amount of calculations still decreased.
However, in no case do not use this technique when adding and subtracting fractions! Yes, sometimes there are similar numbers that you just want to reduce. Here, look:
You can't do that!
The error occurs due to the fact that when adding a fraction, the sum appears in the numerator of a fraction, and not the product of numbers. Therefore, it is impossible to apply the main property of a fraction, since this property deals specifically with the multiplication of numbers.
There is simply no other reason to reduce fractions, so the correct solution to the previous problem looks like this:
Correct solution:
As you can see, the correct answer turned out to be not so beautiful. In general, be careful.
Lesson contentAdding fractions with the same denominators
Adding fractions is of two types:
- Adding fractions with the same denominators;
- Adding fractions with different denominators.
First, we will study the addition of fractions with the same denominators. Everything is simple here. To add fractions with the same denominators, you need to add their numerators, and leave the denominator unchanged.
For example, let's add fractions and . We add the numerators, and leave the denominator unchanged:

This example can be easily understood if we think of a pizza that is divided into four parts. If you add pizza to pizza, you get pizza:

Example 2 Add fractions and .

The answer is an improper fraction. If the end of the task comes, then it is customary to get rid of improper fractions. To get rid of an improper fraction, you need to select the whole part in it. In our case, the whole part stands out easily - two divided by two will be one:

This example can be easily understood if we think of a pizza that is divided into two parts. If you add more pizzas to the pizza, you get one whole pizza:

Example 3. Add fractions and .
Again, add the numerators, and leave the denominator unchanged:
![]()
This example can be easily understood if we think of a pizza that is divided into three parts. If you add more pizzas to pizza, you get pizzas:

Example 4 Find the value of an expression 
This example is solved in exactly the same way as the previous ones. The numerators must be added and the denominator left unchanged:
Let's try to depict our solution using a picture. If you add pizzas to a pizza and add more pizzas, you get 1 whole pizza and more pizzas.

As you can see, adding fractions with the same denominators is not difficult. It is enough to understand the following rules:
- To add fractions with the same denominators, you need to add their numerators, and leave the denominator unchanged;
Adding fractions with different denominators
Now we will learn how to add fractions with different denominators. When adding fractions, the denominators of those fractions must be the same. But they are not always the same.
For example, fractions can be added because they have the same denominators.
But fractions cannot be added at once, because these fractions have different denominators. In such cases, fractions must be reduced to the same (common) denominator.
There are several ways to reduce fractions to the same denominator. Today we will consider only one of them, since the rest of the methods may seem complicated for a beginner.
The essence of this method lies in the fact that first (LCM) of the denominators of both fractions is sought. Then the LCM is divided by the denominator of the first fraction and the first additional factor is obtained. They do the same with the second fraction - the LCM is divided by the denominator of the second fraction and the second additional factor is obtained.
Then the numerators and denominators of the fractions are multiplied by their additional factors. As a result of these actions, fractions that had different denominators turn into fractions that have the same denominators. And we already know how to add such fractions.
Example 1. Add fractions and
First of all, we find the least common multiple of the denominators of both fractions. The denominator of the first fraction is the number 3, and the denominator of the second fraction is the number 2. The least common multiple of these numbers is 6
LCM (2 and 3) = 6
Now back to fractions and . First, we divide the LCM by the denominator of the first fraction and get the first additional factor. LCM is the number 6, and the denominator of the first fraction is the number 3. Divide 6 by 3, we get 2.
The resulting number 2 is the first additional factor. We write it down to the first fraction. To do this, we make a small oblique line above the fraction and write down the found additional factor above it:
We do the same with the second fraction. We divide the LCM by the denominator of the second fraction and get the second additional factor. LCM is the number 6, and the denominator of the second fraction is the number 2. Divide 6 by 2, we get 3.
The resulting number 3 is the second additional factor. We write it to the second fraction. Again, we make a small oblique line above the second fraction and write the found additional factor above it:
Now we are all set to add. It remains to multiply the numerators and denominators of fractions by their additional factors:

Look closely at what we have come to. We came to the conclusion that fractions that had different denominators turned into fractions that had the same denominators. And we already know how to add such fractions. Let's complete this example to the end:

Thus the example ends. To add it turns out.
Let's try to depict our solution using a picture. If you add pizzas to a pizza, you get one whole pizza and another sixth of a pizza:

Reduction of fractions to the same (common) denominator can also be depicted using a picture. Bringing the fractions and to a common denominator, we get the fractions and . These two fractions will be represented by the same slices of pizzas. The only difference will be that this time they will be divided into equal shares (reduced to the same denominator).

The first drawing shows a fraction (four pieces out of six) and the second picture shows a fraction (three pieces out of six). Putting these pieces together we get (seven pieces out of six). This fraction is incorrect, so we have highlighted the integer part in it. The result was (one whole pizza and another sixth pizza).
Note that we have painted this example in too much detail. In educational institutions it is not customary to write in such a detailed manner. You need to be able to quickly find the LCM of both denominators and additional factors to them, as well as quickly multiply the additional factors found by your numerators and denominators. While at school, we would have to write this example as follows:

But there is also the other side of the coin. If detailed notes are not made at the first stages of studying mathematics, then questions of the kind “Where does that number come from?”, “Why do fractions suddenly turn into completely different fractions? «.
To make it easier to add fractions with different denominators, you can use the following step-by-step instructions:
- Find the LCM of the denominators of fractions;
- Divide the LCM by the denominator of each fraction and get an additional multiplier for each fraction;
- Multiply the numerators and denominators of fractions by their additional factors;
- Add fractions that have the same denominators;
- If the answer turned out to be an improper fraction, then select its whole part;
Example 2 Find the value of an expression  .
.
Let's use the instructions above.
Step 1. Find the LCM of the denominators of fractions
Find the LCM of the denominators of both fractions. The denominators of the fractions are the numbers 2, 3 and 4
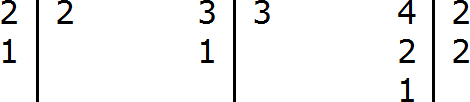
Step 2. Divide the LCM by the denominator of each fraction and get an additional multiplier for each fraction
Divide the LCM by the denominator of the first fraction. LCM is the number 12, and the denominator of the first fraction is the number 2. Divide 12 by 2, we get 6. We got the first additional factor 6. We write it over the first fraction:
Now we divide the LCM by the denominator of the second fraction. LCM is the number 12, and the denominator of the second fraction is the number 3. Divide 12 by 3, we get 4. We got the second additional factor 4. We write it over the second fraction:
Now we divide the LCM by the denominator of the third fraction. LCM is the number 12, and the denominator of the third fraction is the number 4. Divide 12 by 4, we get 3. We got the third additional factor 3. We write it over the third fraction:
Step 3. Multiply the numerators and denominators of fractions by your additional factors
We multiply the numerators and denominators by our additional factors:
Step 4. Add fractions that have the same denominators
We came to the conclusion that fractions that had different denominators turned into fractions that have the same (common) denominators. It remains to add these fractions. Add up:

The addition didn't fit on one line, so we moved the remaining expression to the next line. This is allowed in mathematics. When an expression does not fit on one line, it is carried over to the next line, and it is necessary to put an equal sign (=) at the end of the first line and at the beginning of a new line. The equal sign on the second line indicates that this is a continuation of the expression that was on the first line.
Step 5. If the answer turned out to be an improper fraction, then select the whole part in it
Our answer is an improper fraction. We must single out the whole part of it. We highlight:

Got an answer
Subtraction of fractions with the same denominators
There are two types of fraction subtraction:
- Subtraction of fractions with the same denominators
- Subtraction of fractions with different denominators
First, let's learn how to subtract fractions with the same denominators. Everything is simple here. To subtract another from one fraction, you need to subtract the numerator of the second fraction from the numerator of the first fraction, and leave the denominator the same.
For example, let's find the value of the expression . To solve this example, it is necessary to subtract the numerator of the second fraction from the numerator of the first fraction, and leave the denominator unchanged. Let's do this:

This example can be easily understood if we think of a pizza that is divided into four parts. If you cut pizzas from a pizza, you get pizzas:

Example 2 Find the value of the expression .
Again, from the numerator of the first fraction, subtract the numerator of the second fraction, and leave the denominator unchanged:

This example can be easily understood if we think of a pizza that is divided into three parts. If you cut pizzas from a pizza, you get pizzas:

Example 3 Find the value of an expression 
This example is solved in exactly the same way as the previous ones. From the numerator of the first fraction, you need to subtract the numerators of the remaining fractions:

As you can see, there is nothing complicated in subtracting fractions with the same denominators. It is enough to understand the following rules:
- To subtract another from one fraction, you need to subtract the numerator of the second fraction from the numerator of the first fraction, and leave the denominator unchanged;
- If the answer turned out to be an improper fraction, then you need to select the whole part in it.
Subtraction of fractions with different denominators
For example, a fraction can be subtracted from a fraction, since these fractions have the same denominators. But a fraction cannot be subtracted from a fraction, because these fractions have different denominators. In such cases, fractions must be reduced to the same (common) denominator.
The common denominator is found according to the same principle that we used when adding fractions with different denominators. First of all, find the LCM of the denominators of both fractions. Then the LCM is divided by the denominator of the first fraction and the first additional factor is obtained, which is written over the first fraction. Similarly, the LCM is divided by the denominator of the second fraction and a second additional factor is obtained, which is written over the second fraction.
The fractions are then multiplied by their additional factors. As a result of these operations, fractions that had different denominators turn into fractions that have the same denominators. And we already know how to subtract such fractions.
Example 1 Find the value of an expression:
These fractions have different denominators, so you need to bring them to the same (common) denominator.
First, we find the LCM of the denominators of both fractions. The denominator of the first fraction is the number 3, and the denominator of the second fraction is the number 4. The least common multiple of these numbers is 12
LCM (3 and 4) = 12
Now back to fractions and
Let's find an additional factor for the first fraction. To do this, we divide the LCM by the denominator of the first fraction. LCM is the number 12, and the denominator of the first fraction is the number 3. Divide 12 by 3, we get 4. We write the four over the first fraction:
We do the same with the second fraction. We divide the LCM by the denominator of the second fraction. LCM is the number 12, and the denominator of the second fraction is the number 4. Divide 12 by 4, we get 3. Write a triple over the second fraction:
Now we are all set for subtraction. It remains to multiply the fractions by their additional factors:

We came to the conclusion that fractions that had different denominators turned into fractions that had the same denominators. And we already know how to subtract such fractions. Let's complete this example to the end:

Got an answer
Let's try to depict our solution using a picture. If you cut pizzas from a pizza, you get pizzas.

This is the detailed version of the solution. Being at school, we would have to solve this example in a shorter way. Such a solution would look like this:

Reduction of fractions and to a common denominator can also be depicted using a picture. Bringing these fractions to a common denominator, we get the fractions and . These fractions will be represented by the same pizza slices, but this time they will be divided into the same fractions (reduced to the same denominator):

The first drawing shows a fraction (eight pieces out of twelve), and the second picture shows a fraction (three pieces out of twelve). By cutting off three pieces from eight pieces, we get five pieces out of twelve. The fraction describes these five pieces.
Example 2 Find the value of an expression 
These fractions have different denominators, so you first need to bring them to the same (common) denominator.
Find the LCM of the denominators of these fractions.
The denominators of the fractions are the numbers 10, 3 and 5. The least common multiple of these numbers is 30
LCM(10, 3, 5) = 30
Now we find additional factors for each fraction. To do this, we divide the LCM by the denominator of each fraction.
Let's find an additional factor for the first fraction. LCM is the number 30, and the denominator of the first fraction is the number 10. Divide 30 by 10, we get the first additional factor 3. We write it over the first fraction:
Now we find an additional factor for the second fraction. Divide the LCM by the denominator of the second fraction. LCM is the number 30, and the denominator of the second fraction is the number 3. Divide 30 by 3, we get the second additional factor 10. We write it over the second fraction:
Now we find an additional factor for the third fraction. Divide the LCM by the denominator of the third fraction. LCM is the number 30, and the denominator of the third fraction is the number 5. Divide 30 by 5, we get the third additional factor 6. We write it over the third fraction:
Now everything is ready for subtraction. It remains to multiply the fractions by their additional factors:
We came to the conclusion that fractions that had different denominators turned into fractions that have the same (common) denominators. And we already know how to subtract such fractions. Let's finish this example.
The continuation of the example will not fit on one line, so we move the continuation to the next line. Don't forget about the equal sign (=) on the new line:

The answer turned out to be a correct fraction, and everything seems to suit us, but it is too cumbersome and ugly. We should make it easier. What can be done? You can reduce this fraction.
To reduce a fraction, you need to divide its numerator and denominator by (gcd) the numbers 20 and 30.
So, we find the GCD of the numbers 20 and 30:

Now we return to our example and divide the numerator and denominator of the fraction by the found GCD, that is, by 10

Got an answer
Multiplying a fraction by a number
To multiply a fraction by a number, you need to multiply the numerator of the given fraction by this number, and leave the denominator unchanged.
Example 1. Multiply the fraction by the number 1.
Multiply the numerator of the fraction by the number 1
![]()
The entry can be understood as taking half 1 time. For example, if you take pizza 1 time, you get pizza

From the laws of multiplication, we know that if the multiplicand and the multiplier are interchanged, then the product will not change. If the expression is written as , then the product will still be equal to . Again, the rule for multiplying an integer and a fraction works:
![]()
This entry can be understood as taking half of the unit. For example, if there is 1 whole pizza and we take half of it, then we will have pizza:

Example 2. Find the value of an expression
Multiply the numerator of the fraction by 4
![]()
The answer is an improper fraction. Let's take a whole part of it:
![]()
The expression can be understood as taking two quarters 4 times. For example, if you take pizzas 4 times, you get two whole pizzas.

And if we swap the multiplicand and the multiplier in places, we get the expression. It will also be equal to 2. This expression can be understood as taking two pizzas from four whole pizzas:

A number that is multiplied by a fraction and the denominator of the fraction are resolved if they have a common divisor greater than one.
For example, an expression can be evaluated in two ways.
First way. Multiply the number 4 by the numerator of the fraction, and leave the denominator of the fraction unchanged:
![]()
Second way. The quadruple being multiplied and the quadruple in the denominator of the fraction can be reduced. You can reduce these fours by 4, since the greatest common divisor for two fours is the four itself:

We got the same result 3. After reducing the fours, new numbers are formed in their place: two ones. But multiplying one with a triple, and then dividing by one does not change anything. Therefore, the solution can be written shorter:
The reduction can be performed even when we decided to use the first method, but at the stage of multiplying the number 4 and the numerator 3, we decided to use the reduction:

But for example, the expression can only be calculated in the first way - multiply 7 by the denominator of the fraction, and leave the denominator unchanged:
![]()
This is due to the fact that the number 7 and the denominator of the fraction do not have a common divisor greater than one, and, accordingly, are not reduced.
Some students mistakenly abbreviate the number being multiplied and the numerator of the fraction. You can't do this. For example, the following entry is not correct:
The reduction of the fraction implies that and numerator and denominator will be divided by the same number. In the situation with the expression, the division is performed only in the numerator, since writing this is the same as writing . We see that the division is performed only in the numerator, and no division occurs in the denominator.
Multiplication of fractions
To multiply fractions, you need to multiply their numerators and denominators. If the answer is an improper fraction, you need to select the whole part in it.
Example 1 Find the value of the expression .
![]()
Got an answer. It is desirable to reduce this fraction. The fraction can be reduced by 2. Then the final solution will take the following form:

The expression can be understood as taking a pizza from half a pizza. Let's say we have half a pizza:
How to take two-thirds from this half? First you need to divide this half into three equal parts:

And take two from these three pieces:

We'll get pizza. Remember what a pizza looks like divided into three parts:

One slice from this pizza and the two slices we took will have the same dimensions:

In other words, we are talking about the same pizza size. Therefore, the value of the expression is

Example 2. Find the value of an expression
Multiply the numerator of the first fraction by the numerator of the second fraction, and the denominator of the first fraction by the denominator of the second fraction:

The answer is an improper fraction. Let's take a whole part of it:
![]()
Example 3 Find the value of an expression
Multiply the numerator of the first fraction by the numerator of the second fraction, and the denominator of the first fraction by the denominator of the second fraction:
![]()
The answer turned out to be a correct fraction, but it will be good if it is reduced. To reduce this fraction, you need to divide the numerator and denominator of this fraction by the greatest common divisor (GCD) of the numbers 105 and 450.
So, let's find the GCD of the numbers 105 and 450:

Now we divide the numerator and denominator of our answer to the GCD that we have now found, that is, by 15
Representing an integer as a fraction
Any whole number can be represented as a fraction. For example, the number 5 can be represented as . From this, the five will not change its meaning, since the expression means “the number five divided by one”, and this, as you know, is equal to five:
Reverse numbers
Now we will get acquainted with a very interesting topic in mathematics. It's called "reverse numbers".
Definition. Reverse to numbera is the number that, when multiplied bya gives a unit.
Let's substitute in this definition instead of a variable a number 5 and try to read the definition:
Reverse to number 5 is the number that, when multiplied by 5 gives a unit.
Is it possible to find a number that, when multiplied by 5, gives one? It turns out you can. Let's represent five as a fraction:
Then multiply this fraction by itself, just swap the numerator and denominator. In other words, let's multiply the fraction by itself, only inverted:
What will be the result of this? If we continue to solve this example, we get one:
![]()
This means that the inverse of the number 5 is the number, since when 5 is multiplied by one, one is obtained.
The reciprocal can also be found for any other integer.
You can also find the reciprocal for any other fraction. To do this, it is enough to turn it over.
Division of a fraction by a number
Let's say we have half a pizza:
Let's divide it equally between two. How many pizzas will each get?

It can be seen that after splitting half of the pizza, two equal pieces were obtained, each of which makes up a pizza. So everyone gets a pizza.
