In what cases the probability theorem is added. The addition theorem for the probabilities of incompatible events

Theorems of addition and multiplication of probabilities.

The theorem of addition of probabilities of two events. The probability of the sum of two events is equal to the sum of the probabilities of these events without the probability of their joint occurrence:

P(A+B)=P(A)+P(B)-P(AB).

The theorem of addition of probabilities of two incompatible events. The probability of the sum of two incompatible events is equal to the sum of the probabilities of these:

P(A+B)=P(A)+P(B).

Example 2.16. The shooter shoots at a target divided into 3 areas. The probability of hitting the first area is 0.45, the second - 0.35. Find the probability that the shooter will hit either the first or the second area with one shot.

Solution.

Events A- "the shooter hit the first area" and IN- “the shooter hit the second area” - are inconsistent (hitting in one area excludes getting into another), so the addition theorem is applicable.

The desired probability is equal to:

P(A+B)=P(A)+P(B)= 0,45+ 0,35 = 0,8.

Addition theorem P incompatible events. The probability of the sum of n incompatible events is equal to the sum of the probabilities of these:

P (A 1 + A 2 + ... + A p) \u003d P (A 1) + P (A 2) + ... + P (A p).

The sum of the probabilities of opposite events is equal to one:

Event Probability IN assuming an event has occurred A, is called the conditional probability of the event IN and is marked like this: P(B/A), or R A (B).

. The probability of the product of two events is equal to the product of the probability of one of them by the conditional probability of the other, provided that the first event occurred:

P(AB)=P(A)P A(B).

Event IN does not depend on the event A, If

P A (B) \u003d P (B),

those. event probability IN does not depend on whether the event occurred A.

The theorem of multiplication of probabilities of two independent events.The probability of the product of two independent events is equal to the product of their probabilities:

P(AB)=P(A)P(B).

Example 2.17. The probabilities of hitting the target when firing the first and second guns are respectively equal: p 1 = 0,7; p 2= 0.8. Find the probability of hitting with one volley (from both guns) by at least one of the guns.

Solution.

The probability of hitting the target by each of the guns does not depend on the result of firing from the other gun, so the events A- "First gun hit" and IN– “second gun hit” are independent.

Event Probability AB- "both guns hit":

Desired probability

P (A + B) \u003d P (A) + P (B) - P (AB)= 0,7 + 0,8 – 0,56 = 0,94.

Probability multiplication theorem P events.The probability of a product of n events is equal to the product of one of them by the conditional probabilities of all the others, calculated under the assumption that all previous events have occurred:

Example 2.18. An urn contains 5 white, 4 black and 3 blue balls. Each test consists in the fact that one ball is drawn at random without returning it back. Find the probability that a white ball will appear on the first trial (event A), a black ball on the second trial (event B), and a blue ball on the third trial (event C).

Solution.

Probability of a white ball appearing in the first trial:

The probability of a black ball appearing in the second trial, calculated assuming that a white ball appeared in the first trial, i.e. the conditional probability:

The probability of a blue ball appearing in the third trial, calculated assuming that a white ball appeared in the first trial and a black one in the second, i.e. the conditional probability:

The desired probability is equal to:

Probability multiplication theorem P independent events.The probability of a product of n independent events is equal to the product of their probabilities:

P (A 1 A 2 ... A p) \u003d P (A 1) P (A 2) ... P (A p).

The probability that at least one of the events will occur. The probability of occurrence of at least one of the events A 1 , A 2 , ..., A p, independent in the aggregate, is equal to the difference between unity and the product of the probabilities of opposite events:

.

Example 2.19. The probabilities of hitting the target when firing from three guns are as follows: p 1 = 0,8; p 2 = 0,7;p 3= 0.9. Find the probability of at least one hit (event A) with one salvo from all guns.

Solution.

The probability of hitting the target by each of the guns does not depend on the results of firing from other guns, so the events under consideration A 1(hit by the first gun), A 2(hit by the second gun) and A 3(hit of the third gun) are independent in the aggregate.

Probabilities of events opposite to events A 1, A 2 And A 3(i.e. miss probabilities), respectively, are equal to:

, , .

The desired probability is equal to:

If independent events A 1, A 2, ..., A p have the same probability R, then the probability of occurrence of at least one of these events is expressed by the formula:

Р(А)= 1 – q n ,

Where q=1-p

2.7. Total Probability Formula. Bayes formula.

Let the event A can occur if one of the incompatible events occurs N 1, N 2, ..., N p, forming a complete group of events. Since it is not known in advance which of these events will occur, they are called hypotheses.

Probability of an event occurring A calculated by total probability formula:

P (A) \u003d P (N 1) P (A / N 1) + P (N 2) P (A / N 2) + ... + P (N p) P (A / N p).

Let us assume that an experiment has been carried out, as a result of which the event A happened. Conditional event probabilities N 1, N 2, ..., N p regarding the event A determined Bayes formulas:

,

Example 2.20. In a group of 20 students who came to the exam, 6 are excellent, 8 are good, 4 are satisfactory and 2 are poorly prepared. There are 30 questions in the exam papers. A well-prepared student can answer all 30 questions, a well-prepared student can answer 24, a satisfactory student can answer 15, and a poor student can answer 7.

A randomly selected student answered three random questions. Find the probability that this student is prepared: a) excellent; b) bad.

Solution.

Hypotheses - "the student is well prepared";

– “the student is well prepared”;

– “the student is prepared satisfactorily”;

- "the student is poorly prepared."

Before experience:

; ; ; ;

7. What is called a complete group of events?

8. What events are called equally likely? Give examples of such events.

9. What is called an elementary outcome?

10. What outcomes do I call favorable to this event?

11. What operations can be performed on events? Give them definitions. How are they designated? Give examples.

12. What is called probability?

13. What is the probability of a certain event?

14. What is the probability of an impossible event?

15. What are the limits of probability?

16. How is the geometric probability on the plane determined?

17. How is probability defined in space?

18. How is the probability on a straight line determined?

19. What is the probability of the sum of two events?

20. What is the probability of the sum of two incompatible events?

21. What is the probability of the sum of n incompatible events?

22. What is the conditional probability? Give an example.

23. Formulate the probabilities multiplication theorem.

24. How to find the probability of occurrence of at least one of the events?

25. What events are called hypotheses?

26. When are the total probability formula and Bayes formulas applied?

Addition theorem

Consider incompatible random events.

It is known that incompatible random events $A$ and $B$ in the same trial have probabilities $P\left(A\right)$ and $P\left(B\right)$ respectively. Let us find the probability of the sum $A+B$ of these events, i.e. the probability of occurrence of at least one of them.

Suppose that in this test the number of all equally possible elementary events is $n$. Of these, events $A$ and $B$ are favored by $m_(A)$ and $m_(B)$ elementary events, respectively. Since the events $A$ and $B$ are incompatible, the event $A+B$ is favored by $m_(A) +m_(B)$ elementary events. We have $P\left(A+B\right)=\frac(m_(A) +m_(B) )(n) =\frac(m_(A) )(n) +\frac(m_(B) ) (n) =P\left(A\right)+P\left(B\right)$.

Theorem 1

The probability of the sum of two incompatible events is equal to the sum of their probabilities.

Note 1

Consequence 1. The probability of the sum of any number of incompatible events is equal to the sum of the probabilities of these events.

Consequence 2. The sum of the probabilities of a complete group of incompatible events (the sum of the probabilities of all elementary events) is equal to one.

Consequence 3. The sum of the probabilities of opposite events is equal to one, since they form a complete group of incompatible events.

Example 1

The probability that it will never rain in the city for some time is $p=0.7$. Find the probability $q$ that during the same time it will rain in the city at least once.

The events "for some time it never rained in the city" and "for some time it rained in the city at least once" are opposite. Therefore $p+q=1$, whence $q=1-p=1-0.7=0.3$.

Consider joint random events.

It is known that joint random events $A$ and $B$ in the same trial have probabilities $P\left(A\right)$ and $P\left(B\right)$ respectively. Let us find the probability of the sum $A+B$ of these events, i.e. the probability of occurrence of at least one of them.

Suppose that in this test the number of all equally possible elementary events is $n$. Of these, events $A$ and $B$ are favored by $m_(A)$ and $m_(B)$ elementary events, respectively. Since the events $A$ and $B$ are joint, then out of the total number of $m_(A) +m_(B) $ elementary events, a certain number $m_(AB) $ favors both the event $A$ and the event $B$, that is, their joint occurrence (the product of events $A\cdot B$). This quantity $m_(AB)$ entered both $m_(A)$ and $m_(B)$. So event $A+B$ is favored by $m_(A) +m_(B) -m_(AB) $ elementary events. We have: $P\left(A+B\right)=\frac(m_(A) +m_(B) -m_(AB) )(n) =\frac(m_(A) )(n) +\frac (m_(B) )(n) -\frac(m_(AB) )(n) =P\left(A\right)+P\left(B\right)-P\left(A\cdot B\right )$.

Theorem 2

The probability of the sum of two joint events is equal to the sum of the probabilities of these events minus the probability of their product.

Comment. If the events $A$ and $B$ are incompatible, then their product $A\cdot B$ is an impossible event whose probability is $P\left(A\cdot B\right)=0$. Therefore, the formula for adding the probabilities of incompatible events is a special case of the formula for adding the probabilities of joint events.

Example 2

Find the probability that when two dice are thrown at the same time, the number 5 will come up at least once.

When throwing two dice at the same time, the number of all equally possible elementary events is equal to $n=36$, since six digits of the second die can fall on each digit of the first dice. Of these, the event $A$ - the number 5 rolled on the first die - occurs 6 times, the event $B$ - the number 5 rolled on the second die - also occurs 6 times. Of all twelve times, the number 5 appears once on both dice. So $P\left(A+B\right)=\frac(6)(36) +\frac(6)(36) -\frac(1)(36) =\frac(11)(36) $.

Probability multiplication theorem

Consider independent events.

Events $A$ and $B$ that occur in two successive trials are called independent if the probability of occurrence of event $B$ does not depend on whether event $A$ took place or did not take place.

For example, suppose there are 2 white and 2 black balls in an urn. The test is to extract the ball. The event $A$ is "a white ball is drawn in the first trial". Probability $P\left(A\right)=\frac(1)(2) $. After the first test, the ball was put back and a second test was carried out. Event $B$ -- ``white ball drawn in second trial''. Probability $P\left(B\right)=\frac(1)(2) $. The probability $P\left(B\right)$ does not depend on whether the event $A$ took place or not, hence the events $A$ and $B$ are independent.

It is known that independent random events $A$ and $B$ of two consecutive trials have probabilities $P\left(A\right)$ and $P\left(B\right)$ respectively. Let us find the probability of the product $A\cdot B$ of these events, that is, the probability of their joint occurrence.

Suppose that in the first trial the number of all equally possible elementary events is $n_(1) $. Of these, $A$ is favored by $m_(1)$ elementary events. Let us also assume that in the second test the number of all equally possible elementary events is $n_(2) $. Of these, event $B$ is favored by $m_(2)$ elementary events. Now consider a new elementary event, which consists in the successive occurrence of events from the first and second trials. The total number of such equally probable elementary events is equal to $n_(1) \cdot n_(2) $. Since the events $A$ and $B$ are independent, from this number the joint occurrence of the event $A$ and the event $B$ (the products of the events $A\cdot B$) is favored by $m_(1) \cdot m_(2) $ events . We have: $P\left(A\cdot B\right)=\frac(m_(1) \cdot m_(2) )(n_(1) \cdot n_(2) ) =\frac(m_(1) ) (n_(1) ) \cdot \frac(m_(2) )(n_(2) ) =P\left(A\right)\cdot P\left(B\right)$.

Theorem 3

The probability of the product of two independent events is equal to the product of the probabilities of these events.

Consider dependent events.

In two consecutive trials, events $A$ and $B$ occur. An event $B$ is said to be dependent on the event $A$ if the probability of occurrence of the event $B$ depends on whether the event $A$ took place or not. Then the probability of the event $B$, which was calculated under the condition that the event $A$ took place, is called the conditional probability of the event $B$ under the condition $A$ and is denoted by $P\left(B/A\right)$.

For example, suppose there are 2 white and 2 black balls in an urn. The test is the extraction of the ball. The event $A$ is "a white ball is drawn in the first trial". Probability $P\left(A\right)=\frac(1)(2) $. After the first test, the ball is not put back and the second test is performed. Event $B$ -- ``white ball drawn in second trial''. If a white ball was drawn in the first trial, then the probability is $P\left(B/A\right)=\frac(1)(3) $. If a black ball was drawn in the first trial, then the probability is $P\left(B/\overline(A)\right)=\frac(2)(3) $. Thus, the probability of the event $B$ depends on whether the event $A$ took place or not, therefore, the event $B$ depends on the event $A$.

Assume that events $A$ and $B$ occur in two consecutive trials. It is known that the event $A$ has the probability of occurrence $P\left(A\right)$. It is also known that the event $B$ is dependent on the event $A$ and its conditional probability under condition $A$ is equal to $P\left(B/A\right)$.

Theorem 4

The probability of the product of the event $A$ and the event $B$ dependent on it, that is, the probability of their joint occurrence, can be found by the formula $P\left(A\cdot B\right)=P\left(A\right)\cdot P\left(B/A\right)$.

The symmetric formula $P\left(A\cdot B\right)=P\left(B\right)\cdot P\left(A/B\right)$ is also valid, where the event $A$ is assumed to be dependent on the event $ B$.

For the conditions of the last example, we find the probability that the white ball will be drawn in both trials. Such an event is a product of the events $A$ and $B$. Its probability is $P\left(A\cdot B\right)=P\left(A\right)\cdot P\left(B/A\right)=\frac(1)(2) \cdot \frac( 1)(3) =\frac(1)(6) $.

Educational Institution "Belarusian State

agricultural Academy"

Department of Higher Mathematics

ADDITION AND MULTIPLICATION OF PROBABILITIES. REPEATED INDEPENDENT TESTS

Lecture for students of the Faculty of Land Management

distance learning

Gorki, 2012

Addition and multiplication of probabilities. Repeated

independent tests

    Addition of probabilities

The sum of two joint events A And IN called an event WITH, consisting in the occurrence of at least one of the events A or IN. Similarly, the sum of several joint events is an event consisting in the occurrence of at least one of these events.

The sum of two disjoint events A And IN called an event WITH, consisting in the occurrence or event A, or events IN. Similarly, the sum of several incompatible events is an event consisting in the occurrence of any one of these events.

The theorem of addition of probabilities of incompatible events is valid: the probability of the sum of two incompatible events is equal to the sum of the probabilities of these events , i.e. . This theorem can be extended to any finite number of incompatible events.

From this theorem follows:

the sum of the probabilities of events forming a complete group is equal to one;

the sum of the probabilities of opposite events is equal to one, i.e.
.

Example 1 . A box contains 2 white, 3 red and 5 blue balls. The balls are shuffled and one is drawn at random. What is the probability that the ball is colored?

Solution . Let's denote the events:

A=(color ball removed);

B=(white ball drawn);

C=(red ball drawn);

D=(blue ball removed).

Then A= C+ D. Since the events C, D are incompatible, then we use the theorem of addition of probabilities of incompatible events: .

Example 2 . An urn contains 4 white balls and 6 black balls. 3 balls are drawn at random from the urn. What is the probability that they are all the same color?

Solution . Let's denote the events:

A\u003d (balls of the same color are taken out);

B\u003d (white balls are taken out);

C= (black balls are taken out).

Because A= B+ C and events IN And WITH are incompatible, then by the theorem of addition of probabilities of incompatible events
. Event Probability IN is equal to
, Where
4,

. Substitute k And n into the formula and get
Similarly, we find the probability of an event WITH:
, Where
,
, i.e.
. Then
.

Example 3 . From a deck of 36 cards, 4 cards are drawn at random. Find the probability that there will be at least three aces among them.

Solution . Let's denote the events:

A\u003d (among the drawn cards there are at least three aces);

B\u003d (among the drawn cards there are three aces);

C= (among the drawn cards there are four aces).

Because A= B+ C, and the events IN And WITH inconsistent, then
. Let's find the probabilities of events IN And WITH:


,
. Therefore, the probability that among the drawn cards there are at least three aces is equal to

0.0022.

    Probability multiplication

work two events A And IN called an event WITH, consisting in the joint occurrence of these events:
. This definition extends to any finite number of events.

The two events are called independent if the probability of occurrence of one of them does not depend on whether the other event occurred or not. Events ,, … ,called collectively independent , if the probability of occurrence of each of them does not depend on whether other events occurred or did not occur.

Example 4 . Two arrows shoot at a target. Let's denote the events:

A=(first shooter hit the target);

B= (the second shooter hit the target).

Obviously, the probability of hitting the target by the first shooter does not depend on whether the second shooter hit or missed, and vice versa. Therefore, the events A And IN independent.

The theorem of multiplication of probabilities of independent events is valid: the probability of the product of two independent events is equal to the product of the probabilities of these events : .

This theorem is also valid for n events that are independent in the aggregate: .

Example 5 . Two shooters shoot at the same target. The probability of hitting the first shooter is 0.9, and the second is 0.7. Both shooters fire one shot at the same time. Determine the probability that there will be two hits on the target.

Solution . Let's denote the events:

A

B

C=(both arrows will hit the target).

Because
, and the events A And IN independent, then
, i.e..

Events A And IN called dependent if the probability of occurrence of one of them depends on whether the other event occurred or not. Probability of an event A provided that the event IN it's already here, it's called conditional probability and denoted
or
.

Example 6 . An urn contains 4 white and 7 black balls. Balls are drawn from the urn. Let's denote the events:

A=(white ball removed) ;

B=(black ball removed).

Before you start drawing balls from the urn
. One ball is drawn from the urn and it turns out to be black. Then the probability of the event A after the event IN will be different, equal . This means that the probability of an event A event dependent IN, i.e. these events will be dependent.

The theorem of multiplication of probabilities of dependent events is valid: the probability of the product of two dependent events is equal to the product of the probability of one of them by the conditional probability of the other, calculated on the assumption that the first event has already occurred, i.e. or.

Example 7 . An urn contains 4 white balls and 8 red balls. Two balls are drawn at random from it. Find the probability that both balls are black.

Solution . Let's denote the events:

A=(black ball drawn first);

B=(a black ball is drawn second).

Events A And IN dependent because
, A
. Then
.

Example 8 . Three arrows shoot at the target independently of each other. The probability of hitting the target for the first shooter is 0.5, for the second - 0.6 and for the third - 0.8. Find the probability that two hits will occur if each shooter fires one shot.

Solution . Let's denote the events:

A=(there will be two hits on the target);

B=(first shooter hits the target);

C=(the second shooter will hit the target);

D=(the third shooter will hit the target);

=(the first shooter will not hit the target);

=(the second shooter will not hit the target);

=(the third shooter will not hit the target).

According to the example
,
,
,

,
,
. Since, using the addition theorem for the probabilities of incompatible events and the theorem for multiplying the probabilities of independent events, we get:

Let the events
form a complete group of events of some trial, and the events A can only occur with one of these events. If the probabilities and conditional probabilities of the event are known A, then the probability of event A is calculated by the formula:

or
. This formula is called total probability formula , and the events
hypotheses .

Example 9 . The assembly line receives 700 parts from the first machine and 300 parts from the second. The first machine gives 0.5% rejects, and the second - 0.7%. Find the probability that the item taken is defective.

Solution . Let's denote the events:

A=(the item taken will be defective);

= (the part is made on the first machine);

= (the part is made on the second machine).

The probability that the part was made on the first machine is
. For the second machine
. By the condition, the probability of obtaining a defective part made on the first machine is equal to
. For the second machine, this probability is equal to
. Then the probability that the part taken will be defective is calculated by the total probability formula

If an event is known to have occurred as a result of a test A, then the probability that this event occurred with the hypothesis
, is equal to
, Where
- total probability of the event A. This formula is called Bayes formula and allows you to calculate the probabilities of events
after it became known that the event A has already arrived.

Example 10 . Parts of the same type for cars are produced at two factories and go to the store. The first plant produces 80% of the total number of parts, and the second - 20%. The production of the first plant contains 90% of standard parts, and the second - 95%. The buyer bought one part and it turned out to be standard. Find the probability that this part is made in the second factory.

Solution . Let's denote the events:

A=(purchased a standard part);

= (the part is made at the first factory);

= (the part is made at the second factory).

According to the example
,
,
And
. Calculate the total probability of an event A: 0.91. The probability that the part is manufactured at the second plant is calculated using the Bayes formula:

.

Tasks for independent work

    The probability of hitting the target for the first shooter is 0.8, for the second - 0.7 and for the third - 0.9. The shooters fired one shot. Find the probability that there are at least two hits on the target.

    The repair shop received 15 tractors. It is known that 6 of them need to replace the engine, and the rest - to replace individual components. Three tractors are randomly selected. Find the probability that no more than two selected tractors need an engine replacement.

    The concrete plant produces panels, 80% of which are of the highest quality. Find the probability that out of three randomly selected panels, at least two will be of the highest grade.

    Three workers assemble bearings. The probability that the bearing assembled by the first worker is of the highest quality is 0.7, the second - 0.8, and the third - 0.6. For control, one bearing was taken at random from those assembled by each worker. Find the probability that at least two of them are of the highest quality.

    The probability of winning on a lottery ticket of the first issue is 0.2, the second - 0.3 and the third - 0.25. There is one ticket for each issue. Find the probability that at least two tickets will win.

    The accountant performs calculations using three reference books. The probability that the data of interest to him is in the first directory is 0.6, in the second - 0.7, and in the third - 0.8. Find the probability that the data of interest to the accountant is contained in no more than two directories.

    Three machines make parts. The first automaton produces a part of the highest quality with a probability of 0.9, the second with a probability of 0.7, and the third with a probability of 0.6. One item is taken at random from each machine. Find the probability that at least two of them are of the highest quality.

    The same type of parts are processed on two machines. The probability of manufacturing a non-standard part for the first machine is 0.03, for the second - 0.02. The processed parts are stacked in one place. Among them, 67% are from the first machine, and the rest from the second. A randomly taken part turned out to be standard. Find the probability that it was made on the first machine.

    The workshop received two boxes of the same type of capacitors. The first box contained 20 capacitors, of which 2 were defective. In the second box there are 10 capacitors, of which 3 are faulty. Capacitors were transferred to one box. Find the probability that a capacitor taken at random from the box is good.

    On three machines, the same type of parts are made, which are fed to a common conveyor. Among all the details, 20% from the first machine, 30% from the second and 505 from the third. The probability of manufacturing a standard part on the first machine is 0.8, on the second - 0.6 and on the third - 0.7. The part taken was standard. Find the probability that this part is made on the third machine.

    The picker receives 40% of the parts from the factory for assembly A, and the rest - from the factory IN. The probability that the part from the factory A- the highest quality, equal to 0.8, and from the factory IN– 0.9. The picker randomly took one part and it was not of the highest quality. Find the probability that this part is from the factory IN.

    10 students from the first group and 8 students from the second were selected to participate in student sports competitions. The probability that a student from the first group will get into the national team of the academy is 0.8, and from the second - 0.7. A randomly selected student was selected for the national team. Find the probability that he is from the first group.

    Bernoulli formula

The tests are called independent , if for each of them the event A occurs with the same probability
, regardless of whether this event appeared or did not appear in other trials. Probability of the opposite event in this case equals
.

Example 11 . Throwing a dice n once. Denote the event A= (dropping three points). Probability of an event A in each trial is equal to and does not depend on whether this event occurred or did not occur in other trials. Therefore, these tests are independent. Probability of the opposite event
(not rolling three points) is equal to
.

The probability that in n independent trials, in each of which the probability of an event occurring A is equal to p, the event will occur exactly k times (no matter in what sequence), is calculated by the formula
, Where
. This formula is called Bernoulli formula and it is convenient if the number of trials n is not too large.

Example 12 . The proportion of fetuses infected with the disease in a latent form is 25%. 6 fruits are randomly selected. Find the probability that among the chosen ones there will be: a) exactly 3 infected fetuses; b) no more than two infected fruits.

Solution . According to the example.

a) According to the Bernoulli formula, the probability that exactly three of the six selected fruits will be infected is equal to




0.132.

b) Denote the event A=(infected will be no more than two fetuses). Then . According to the Bernoulli formula:

0.297.

Hence,
0.178+0.356+0.297=0.831.

    Theorems of Laplace and Poisson

The Bernoulli formula is used to find the probability that an event A will come k once a n independent trials and in each trial the probability of an event A constant. For large values ​​of n, calculations using the Bernoulli formula become time-consuming. In this case, to calculate the probability of an event A it is better to use a different formula.

Local Laplace theorem . Let the probability p event A in each test is constant and different from zero and one. Then the probability that the event A comes exactly k times for a sufficiently large number n of trials, is calculated by the formula

, Where
, and the values ​​of the function
are given in the table.

The main properties of the function
are:

Function
is defined and continuous in the interval
.

Function
is positive, i.e.
>0.

Function
even, i.e.
.

Since the function
is even, then the table shows its values ​​​​only for positive values X.

Example 13 . Germination of wheat seeds is 80%. 100 seeds are selected for the experiment. Find the probability that exactly 90 of the selected seeds will germinate.

Solution . According to the example n=100, k=90, p=0.8, q=1-0.8=0.2. Then
. According to the table we find the value of the function
:
. The probability that exactly 90 of the selected seeds will germinate is
0.0044.

When solving practical problems, it becomes necessary to find the probability of an event occurring A at n independent tests at least once and no more once. This problem is solved with the help Laplace integral theorem : Let the probability p event A in each of n independent tests is constant and different from zero and unity. Then the probability that the event will occur is at least once and no more times for a sufficiently large number of tests, is calculated by the formula

Where
,
.

Function
called Laplace function and is not expressed in terms of elementary functions. The values ​​of this function are given in special tables.

The main properties of the function
are:


.

Function
increases in the interval
.


at
.

Function
odd, i.e.
.

Example 14 . The company produces products, of which 13% are not of the highest quality. Determine the probability that in an untested batch of 150 units of the highest quality product there will be at least 125 and at most 135.

Solution . Let's denote . Compute
,

An experiment is being considered E. It is assumed that it can be carried out repeatedly. As a result of the experiment, various events can appear that make up a certain set F. Observed events are divided into three types: reliable, impossible, random.

credible An event is called an event that will definitely occur as a result of an experiment. E. Denoted Ω.

Impossible An event is called an event that is not known to occur as a result of an experiment. E. Designated .

Random an event that may or may not occur as a result of an experiment is called E.

Additional (opposite) event A is called an event, denoted by , that occurs if and only if the event does not occur A.

Sum (combination) events is an event that occurs if and only if at least one of these events occurs (Figure 3.1). Designations .

Figure 3.1

Product (intersection) events is called an event that occurs if and only if all these events occur together (simultaneously) (Figure 3.2). Designations . Obviously, events A and B incompatible , If .

Figure 3.2

Full group of events A set of events is called, the sum of which is a certain event:

Event IN called a special case of an event A, if with the appearance of the event IN event appears A. It is also said that the event IN triggers an event A(Figure 3.3). Designation .

Figure 3.3

Events A And IN called equivalent if they occur or do not occur together during the experiment E. Designation . Obviously, if

Complicated event called an observed event expressed through other events observed in the same experiment using algebraic operations.

The probability of the implementation of a particular complex event is calculated using the formulas for addition and multiplication of probabilities.

Addition theorem

Consequences:

1) in case the events A And IN are inconsistent, the addition theorem takes the form:

2) in the case of three terms, the addition theorem can be written as

3) the sum of the probabilities of mutually opposite events is equal to 1:

The set of events ,, ..., is called full group of events , If

The sum of the probabilities of events forming a complete group is equal to 1:

Probability of an event occurring A provided that the event IN happened, called conditional probability and denote or.

A And IN – dependent events , If .

A And IN – independent events , If .

Probability multiplication theorem

Consequences:

1) for independent events A And IN

2) in the general case, for the product of three events, the probability multiplication theorem has the form:

Problem Solving Samples

Example1 - Three elements are connected in series in an electric circuit, working independently of each other. The failure probabilities of the first, second and third elements are respectively equal to ,,. Find the probability that there is no current in the circuit.

Solution

First way.

Let's designate the events: - in the circuit there was a failure of the first, second and third elements, respectively.

Event A- there will be no current in the circuit (at least one of the elements will fail, since they are connected in series).

Event - current in the circuit (three elements work), . The probability of opposite events is related by formula (3.4). An event is a product of three events that are pairwise independent. By the multiplication theorem for the probabilities of independent events, we obtain

Then the probability of the desired event is .

The second way.

Taking into account the notation adopted earlier, we write the desired event A- at least one of the elements will fail:

Since the terms included in the sum are compatible, we should apply the probability addition theorem in general form for the case of three terms (3.3):

Answer: 0,388.

Tasks for independent solution

1 There are six textbooks on the theory of probability in the reading room, three of which are bound. The librarian took two textbooks at random. Find the probability that both textbooks will be bound.

2 Threads are mixed in a bag, among which 30% are white, and the rest are red. Determine the probabilities that two threads drawn at random will be: of the same color; different colors.

3 The device consists of three elements that work independently. The probabilities of failure-free operation for a certain period of time of the first, second and third elements, respectively, are 0.6; 0.7; 0.8. Find the probabilities that during this time the following will work without fail: only one element; only two elements; all three elements; at least two elements.

4 Three dice are thrown. Find the probabilities of the following events:

a) five points will appear on each side of the dropped out ones;

b) the same number of points will appear on all dropped faces;

c) one point will appear on the two dropped faces, and another number of points will appear on the third face;

d) a different number of points will appear on all the dropped faces.

5 The probability of a shooter hitting the target with one shot is 0.8. How many shots must the shooter fire so that, with a probability less than 0.4, it can be expected that there will be no misses?

6 From the numbers 1, 2, 3, 4, 5, first one is selected, and then from the remaining four - the second digit. All 20 possible outcomes are assumed to be equally likely. Find the probability that an odd digit will be chosen: for the first time; a second time; both times.

7 The probability that a pair of size 46 shoes will be sold again in the men's shoe section of the store is 0.01. How many pairs of shoes must be sold in the store so that with a probability of at least 0.9 it can be expected that at least one pair of size 46 shoes will be sold?

8 There are 10 parts in a box, including two non-standard ones. Find the probability that in six randomly selected parts there will be at most one non-standard one.

9 The technical control department checks products for standardity. The probability that the product is non-standard is 0.1. Find the probability that:

a) of the three products tested, only two will be non-standard;

b) only the fourth checked product in order will be non-standard.

10 32 letters of the Russian alphabet are written on the cards of the split alphabet:

a) Three cards are drawn at random one after the other and placed on the table in the order they appear. Find the probability that the word "world" will turn out;

b) the three cards drawn can be swapped arbitrarily. What is the probability that they can form the word "world"?

11 A fighter attacks a bomber and fires two independent bursts at it. The probability of shooting down a bomber with the first burst is 0.2, and the second is 0.3. If the bomber is not shot down, it fires at the fighter from the stern guns and shoots it down with a probability of 0.25. Find the probability that a bomber or fighter is shot down as a result of air combat.

Homework

1 Total Probability Formula. Bayes formula.

2 solve problems

Task1 . The worker maintains three machines that work independently of each other. The probability that the first machine does not require the attention of a worker within an hour is 0.9, the second - 0.8, the third - 0.85. Find the probability that within an hour at least one machine will require the attention of a worker.

Task2 . The computer center, which must continuously process incoming information, has two computing devices. It is known that each of them has a probability of failure in some time equal to 0.2. It is required to determine the probability:

a) the fact that one of the devices will fail, and the second will be in good order;

b) failure-free operation of each of the devices.

Task3 . Four hunters agreed to shoot at the game in a certain sequence: the next hunter fires only if the previous one misses. The hit probability for the first hunter is 0.6, for the second - 0.7, for the third - 0.8. Find the probability that shots will be fired:

d) four.

Task4 . The part goes through four machining operations. The probability of getting a marriage at the first operation is 0.01, at the second - 0.02, at the third - 0.03, at the fourth - 0.04. Find the probability of receiving a part without defects after four operations, assuming that the events of obtaining defects in individual operations are independent.

The need for operations on probabilities comes when the probabilities of some events are known, and it is necessary to calculate the probabilities of other events that are associated with these events.

Probability addition is used when it is necessary to calculate the probability of a combination or a logical sum of random events.

Sum of events A And B designate A + B or A ∪ B. The sum of two events is an event that occurs if and only if at least one of the events occurs. It means that A + B- an event that occurs if and only if an event occurs during the observation A or event B, or at the same time A And B.

If events A And B are mutually inconsistent and their probabilities are given, then the probability that one of these events will occur as a result of one trial is calculated using the addition of probabilities.

The theorem of addition of probabilities. The probability that one of two mutually incompatible events will occur is equal to the sum of the probabilities of these events:

For example, two shots were fired while hunting. Event A– hitting a duck from the first shot, event IN– hit from the second shot, event ( A+ IN) - hit from the first or second shot or from two shots. So if two events A And IN are incompatible events, then A+ IN- the occurrence of at least one of these events or two events.

Example 1 A box contains 30 balls of the same size: 10 red, 5 blue and 15 white. Calculate the probability that a colored (not white) ball is taken without looking.

Solution. Let's assume that the event A– “the red ball is taken”, and the event IN- "The blue ball is taken." Then the event is “a colored (not white) ball is taken”. Find the probability of an event A:

and events IN:

Events A And IN- mutually incompatible, since if one ball is taken, then balls of different colors cannot be taken. Therefore, we use the addition of probabilities:

The theorem of addition of probabilities for several incompatible events. If the events make up the complete set of events, then the sum of their probabilities is equal to 1:

The sum of the probabilities of opposite events is also equal to 1:

Opposite events form a complete set of events, and the probability of a complete set of events is 1.

The probabilities of opposite events are usually denoted in small letters. p And q. In particular,

from which the following formulas for the probability of opposite events follow:

Example 2 The target in the dash is divided into 3 zones. The probability that a certain shooter will shoot at a target in the first zone is 0.15, in the second zone - 0.23, in the third zone - 0.17. Find the probability that the shooter hits the target and the probability that the shooter misses the target.

Solution: Find the probability that the shooter hits the target:

Find the probability that the shooter misses the target:

More difficult tasks in which you need to apply both addition and multiplication of probabilities - on the page "Various tasks for addition and multiplication of probabilities" .

Addition of probabilities of mutually joint events

Two random events are said to be joint if the occurrence of one event does not preclude the occurrence of a second event in the same observation. For example, when throwing a dice, the event A is considered to be the occurrence of the number 4, and the event IN- dropping an even number. Since the number 4 is an even number, the two events are compatible. In practice, there are tasks for calculating the probabilities of the occurrence of one of the mutually joint events.

The theorem of addition of probabilities for joint events. The probability that one of the joint events will occur is equal to the sum of the probabilities of these events, from which the probability of the common occurrence of both events is subtracted, that is, the product of the probabilities. The formula for the probabilities of joint events is as follows:

Because the events A And IN compatible, event A+ IN occurs if one of three possible events occurs: or AB. According to the theorem of addition of incompatible events, we calculate as follows:

Event A occurs if one of two incompatible events occurs: or AB. However, the probability of occurrence of one event from several incompatible events is equal to the sum of the probabilities of all these events:

Similarly:

Substituting expressions (6) and (7) into expression (5), we obtain the probability formula for joint events:

When using formula (8), it should be taken into account that the events A And IN can be:

  • mutually independent;
  • mutually dependent.

Probability formula for mutually independent events:

Probability formula for mutually dependent events:

If events A And IN are inconsistent, then their coincidence is an impossible case and, thus, P(AB) = 0. The fourth probability formula for incompatible events is as follows:

Example 3 In auto racing, when driving in the first car, the probability of winning, when driving in the second car. Find:

  • the probability that both cars will win;
  • the probability that at least one car will win;

1) The probability that the first car will win does not depend on the result of the second car, so the events A(first car wins) and IN(second car wins) - independent events. Find the probability that both cars win:

2) Find the probability that one of the two cars will win:

More difficult tasks in which you need to apply both addition and multiplication of probabilities - on the page "Various tasks for addition and multiplication of probabilities" .

Solve the problem of addition of probabilities yourself, and then look at the solution

Example 4 Two coins are thrown. Event A- loss of coat of arms on the first coin. Event B- loss of coat of arms on the second coin. Find the probability of an event C = A + B .

Probability multiplication

Multiplication of probabilities is used when the probability of a logical product of events is to be calculated.

In this case, random events must be independent. Two events are said to be mutually independent if the occurrence of one event does not affect the probability of the occurrence of the second event.

Probability multiplication theorem for independent events. The probability of the simultaneous occurrence of two independent events A And IN is equal to the product of the probabilities of these events and is calculated by the formula:

Example 5 The coin is tossed three times in a row. Find the probability that the coat of arms will fall out all three times.

Solution. The probability that the coat of arms will fall on the first toss of a coin, the second time, and the third time. Find the probability that the coat of arms will fall out all three times:

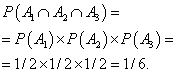
Solve problems for multiplying probabilities yourself, and then look at the solution

Example 6 There is a box with nine new tennis balls. Three balls are taken for the game, after the game they are put back. When choosing balls, they do not distinguish between played and unplayed balls. What is the probability that after three games there will be no unplayed balls in the box?

Example 7 32 letters of the Russian alphabet are written on cut alphabet cards. Five cards are drawn at random, one after the other, and placed on the table in the order in which they appear. Find the probability that the letters will form the word "end".

Example 8 From a full deck of cards (52 sheets), four cards are taken out at once. Find the probability that all four of these cards are of the same suit.

Example 9 The same problem as in example 8, but each card is returned to the deck after being drawn.

More complex tasks, in which you need to apply both addition and multiplication of probabilities, as well as calculate the product of several events - on the page "Various tasks for addition and multiplication of probabilities" .

The probability that at least one of the mutually independent events will occur can be calculated by subtracting the product of the probabilities of opposite events from 1, that is, by the formula:

Example 10 Cargoes are delivered by three modes of transport: river, rail and road transport. The probability that the cargo will be delivered by river transport is 0.82, by rail 0.87, by road 0.90. Find the probability that the goods will be delivered by at least one of the three modes of transport.

RELATED ARTICLES